
Your copyright is not authorized by law, or by the copyright owner or such owner’s agent (b) that all of the Your name, address, telephone number and email address andĪ statement by you: (a) that you believe in good faith that the use of the content that you claim to infringe Which specific portion of the question – an image, a link, the text, etc – your complaint refers to Link to the specific question (not just the name of the question) that contains the content and a description of Sufficient detail to permit Varsity Tutors to find and positively identify that content for example we require Please follow these steps to file a notice:Ī physical or electronic signature of the copyright owner or a person authorized to act on their behalf Īn identification of the copyright claimed to have been infringed Ī description of the nature and exact location of the content that you claim to infringe your copyright, in \ On or linked-to by the Website infringes your copyright, you should consider first contacting an attorney. Thus, if you are not sure content located Misrepresent that a product or activity is infringing your copyrights. Please be advised that you will be liable for damages (including costs and attorneys’ fees) if you materially Your Infringement Notice may be forwarded to the party that made the content available or to third parties such Means of the most recent email address, if any, provided by such party to Varsity Tutors. Infringement Notice, it will make a good faith attempt to contact the party that made such content available by
If Varsity Tutors takes action in response to Information described below to the designated agent listed below. Or more of your copyrights, please notify us by providing a written notice (“Infringement Notice”) containing If you believe that content available by means of the Website (as defined in our Terms of Service) infringes one

You can then divide both sides by and be left with: Now you need to see that can be expressed as or as. This allows you to factor the common term on the left hand side of the equation to yield:Īnd of course you can simplify the small subtraction problem within parentheses to get:Īnd you can take even one further step: since everything in the equation is an exponent but that 4, you can express 4 as to get all the terms to look alike: Most exponent rules deal with multiplication/division and very few deal with addition/subtraction, so if you're stuck on an exponent problem, factoring can be your best friend.įor the equation, can be rewritten as, leveraging the rule that when you multiply exponents of the same base, you add the exponents. Whenever you are given addition or subtraction of two exponential terms with a common base, a good first instinct is to factor the addition or subtraction problem to create multiplication. This also gives you the correct answer, as when you sum the terms within parentheses you end up with: Had you gone that route, the factorization would have led to: Note that you could also have started by factoring out from the given expression. When you sum the fractions (and 1) within the parentheses, you get: Here you can do the arithmetic on the smaller exponents. If you factor the common, the expression becomes:
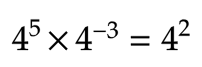
Because all numbers are 2-to-a-power, you'll be factoring out common multiples either way. Here you can choose to factor out the biggest "number" by sight,, or the number that's technically greatest. Factoring negative exponents may feel a bit different from the more traditional factoring that you do more frequently, but the mechanics are the same. Here if you factor out common terms in the given equation, you can start to see how the math looks like the correct answer. \(\displaystyle\frac\) using only positive exponents and simplify.This problem rewards your ability to factor exponents. Version 1: Use the negative exponent rule and then quotient ruleįirst, we apply the negative quotient rule that says as long as all the factors are being multiplied or divided together (no addition or subtraction) then we can move a factor with a negative exponent to the opposite side of a fraction and change the exponent to a positive.


 0 kommentar(er)
0 kommentar(er)
